The 211th day of the year; 211 is a primorial prime,(a prime that is one more, or one less than a primorial can you find the next larger (or smaller) primorial prime?
211 is also the sum of three consecutive primes (67 + 71 + 73)...
There are 211 primes on a 24-hour digital clock. (00:00 - 23:59) *Derek Orr @ Derektionary 211 is the 4th** Euclid number: 1 + product of the first n primes.(after Euclid's method of proving the primes are infinite. most Euclid numbers, unlike 211, are not themselves prime, but are divisible by a prime different than any of the primes in the product n#) (**some would call it the fifth since Euclid seemed to consider 1 as a unit as similar to the primes.)
211 is a prime lucky number, and there are 211 lucky primes less than 10^4 (or 10 ^(2+1+1))*Prime Curios
211 is the concatenation of the smallest one digit prime and the smallest two digit prime, 2, 11.
211 = 3^5 - 2^5, two consecutive fifth powers, it is only the second, following 31, and is the last year date with the property.
Hardy wrote a New Year Resolution in a card to Ramujan to get 211, none out, in a cricket test match at the oval.
A Lazy Caterer number, A Pizza can be cut into 211 pieces with 20 straight cuts.
211 is a repunit in base 14 (111)14^2 + 14 + 1
211 is also SMTP status code for system status.*Wik
211 is an odd number, so it is the difference of two consecutive squares, 106^2 - 105^2 = 211 211 is the minimum sum of any row, column, or diagonal, of a minimum difference (not all rows and columns are the same, but the difference between largest, 213, and smallest, 211, sum is smaller than any other option) prime magic square that contains the 25 primes less than 100 ^Prime Curios
41 79 17 13 61
53 03 83 67 07
59 97 05 23 29
11 31 37 89 43
47 02 71 19 73
211 is the first of fifteen consecutive odd numbers that sum to the cube of 15, 3375
211 is a prime of the form 4k+3. According to Guass' reciprocity law, if two numbers, p and q are in this sequence then there exists a solution to only one of x^2 = p (mod q) or x^2 = q (mod p). 3 is another number in the sequence. Can you find an x^2 so that one of these congruences is true?
And one more from *Prime Curios. If you've ever heard the expression "a month of Sundays," for something that takes a really long time that's 31 Sundays, starting on a Sunday and going for 30 more weeks to end on a Sunday, or 211 days, Sunday to Sunday.
The 212th Day of the Year Besides being the Fahrenheit boiling point of water at sea level, 212 produces a prime of the form k10+k9+...+k2+k+1, when k=212. Edward Shore@edward_shore sent me a note:" That number would be 184,251,916,841,751,188,170,917.") (students might explore different values of k, and different maximum exponents to produce primes..ie when k is 2, then 26 +25+...+22+2+1 is prime)
The smallest even three-digit integer, abc, such that (abc)/(a*b*c) is also prime. [ie 212/(2*1*2)= 53 ]*Prime Curios
212 is a palindrome whose square is also a palindrome, 2122= 44944. It is the last year date for which this is true. It is also a palindrome in base 3(21212) with a copy of it's base 10 representation.
212 a palindrome is the average of two emirps, 113 and 311,
212 is a balanced binary number, with the same number of ones and zeros. 11010100. That means it is one path from (0,0) to (4,4) ,moving only up or right on the lattice. There are 70 such possible paths, How many are always on or above the line y=x?
(212_3) in base three is 23 , also prime in base 7 and base 9 , (212_7) = 107; (212_9) = 173
212 = 14^2 + 4^2, and 54^2 - 52^2
The 213th Day of the Year
213 is a square free number as it has no repeated prime factors. How many days of the year are square free?
For 213, the sum of the digits and the product of the digits are equal, and forms a prime when one is added or subtracted.
The average of the prime factors of 213 is a prime number. [213 = 3*71 and (3+71)/2=37 ]
The square of 213 is a sum of distinct factorials: 2132 = 45369 = 1! + 2! + 3! + 7! + 8!, it is the smallest 3 digit number with this property. (what's next?)
213, like all odd numbers, is the difference of two consecutive squares, 107^2 - 106^2, and because 6*34+9 = 213, 213= 37^2 - 34^2,
a 3x3 magic square with magic constant of 213 using consecutive integers
74 67 72
69 71 73
70 75 68
And with a Hat Tip to John Golden@MathHombre, who designed the google sheet, here is a combination of Latin Squares to form a 3x3 magic square with a constant of 213 (see day 177 for link to his pages)
213^2 = 45369 = 1! + 2! + 3! + 7! + 8!. There are only 11 days that have this property. 213 is the second largest. *HansreudiWidmer.
The 214th Day of the Year
The 11th perfect number 2106 (2107−1) has 214 divisors. Also, 214*412+1 is prime. *Prime Curios
214 is the middle number in a string of three consecutive semiprimes
214 is the last day of the year for which n!!-1 is prime, it is a 205 digit number ending in 25 consecutive nines. (The N!! symbol is often confused with (n!)! in which (3!)! = 6! that it should not be used by teachers. This usage means 214*212*210..*2, and if you wanted every fifth term, you could write n!!!!! which becomes useless at 13, or 43. I much prefer an adjustment of Kramp/ Vandermonde method which I write as \( 214!_2\) but if you only wanted, say the 214 * 212 * 210 * 208, you could add \( 214!_{4|2}\)
Ok, that's a really short list. HELP, what am I missing. Send me your favorite 214 facts.
The 215th Day of the Year
There are 215 sequences of four (not necessarily distinct) integers, counting permutations of order as distinct, such that the sum of their reciprocals is 1. Obviously, one of them is 1/4+1/4+1/4+1/4=1. How many can you find?
If you get stuck, look at the bottom of this Math Day
How many solutions with four distinct integers, not counting permutations?
215 in base six is a repdigit, 215[10] = 555[6]
Lagrange's theorem tells us that each positive integer can be written as a sum of four squares, but Lagrange allowed the use of zeros, such as 12 + 12 + 12 + 02 =3. Allowing only positive integers, there are 57 year days that are not expressible in less than four squares. 215 is the 34th of these year days that is NOT expressible with less than four positive squares. 215 = 12 + 32 + 62 + 132.
The last such number was 207, the next is 220.
You can create more on your own. Take any number in the sequence and multiply it by an odd number and you have another that is in the seque. nce.
In 1937 Lothar Collatz conjectured the process of starting with any number and repeatedly multiplying by 3n+1 if odd, or dividing by two if even in iteration will always lead to one. I mention that here because if you start with 215, it will take 101 operations (a prime number) before you get back to one.
215 = (3!)^3-1 *Wik
Every multiple of 5 greater than 30 is expressible as the difference of the squares of two numbers that differ by 5. (Nice algebra problem for younger students.) 215 = 24^2 - 19^2.
The 213th Day of the Year
213 is a square free number as it has no repeated prime factors. How many days of the year are square free?
For 213, the sum of the digits and the product of the digits are equal, and forms a prime when one is added or subtracted.
The average of the prime factors of 213 is a prime number. [213 = 3*71 and (3+71)/2=37 ]
The square of 213 is a sum of distinct factorials: 2132 = 45369 = 1! + 2! + 3! + 7! + 8!, it is the smallest 3 digit number with this property. (what's next?)
213, like all odd numbers, is the difference of two consecutive squares, 107^2 - 106^2, and because 6*34+9 = 213, 213= 37^2 - 34^2,
a 3x3 magic square with magic constant of 213 using consecutive integers
74 67 72
69 71 73
70 75 68
And with a Hat Tip to John Golden@MathHombre, who designed the google sheet, here is a combination of Latin Squares to form a 3x3 magic square with a constant of 213 (see day 177 for link to his pages)
213^2 = 45369 = 1! + 2! + 3! + 7! + 8!. There are only 11 days that have this property. 213 is the second largest. *HansreudiWidmer.
The 214th Day of the Year
The 11th perfect number 2106 (2107−1) has 214 divisors. Also, 214*412+1 is prime. *Prime Curios
214 is the middle number in a string of three consecutive semiprimes
214 is the last day of the year for which n!!-1 is prime, it is a 205 digit number ending in 25 consecutive nines. (The N!! symbol is often confused with (n!)! in which (3!)! = 6! that it should not be used by teachers. This usage means 214*212*210..*2, and if you wanted every fifth term, you could write n!!!!! which becomes useless at 13, or 43. I much prefer an adjustment of Kramp/ Vandermonde method which I write as \( 214!_2\) but if you only wanted, say the 214 * 212 * 210 * 208, you could add \( 214!_{4|2}\)
Ok, that's a really short list. HELP, what am I missing. Send me your favorite 214 facts.
The 215th Day of the Year
There are 215 sequences of four (not necessarily distinct) integers, counting permutations of order as distinct, such that the sum of their reciprocals is 1. Obviously, one of them is 1/4+1/4+1/4+1/4=1. How many can you find?
If you get stuck, look at the bottom of this Math Day
How many solutions with four distinct integers, not counting permutations?
215 in base six is a repdigit, 215[10] = 555[6]
Lagrange's theorem tells us that each positive integer can be written as a sum of four squares, but Lagrange allowed the use of zeros, such as 12 + 12 + 12 + 02 =3. Allowing only positive integers, there are 57 year days that are not expressible in less than four squares. 215 is the 34th of these year days that is NOT expressible with less than four positive squares. 215 = 12 + 32 + 62 + 132.
The last such number was 207, the next is 220.
You can create more on your own. Take any number in the sequence and multiply it by an odd number and you have another that is in the seque. nce.
In 1937 Lothar Collatz conjectured the process of starting with any number and repeatedly multiplying by 3n+1 if odd, or dividing by two if even in iteration will always lead to one. I mention that here because if you start with 215, it will take 101 operations (a prime number) before you get back to one.
215 = (3!)^3-1 *Wik
Every multiple of 5 greater than 30 is expressible as the difference of the squares of two numbers that differ by 5. (Nice algebra problem for younger students.) 215 = 24^2 - 19^2.
215 is the second (and last) yearday that N^2 - 17 is a square. The next such number is over 4000, but can you find the smaller?
Like all odd numbers, 215 is the difference of two consecutive squares, 108^2 - 107^2 = 215. Because it ends in five (and is bigger than 35) it is also the differences of two squares of numbers that differ by 5, 24^2 - 19^2 = 215
All twin primes after 3 are of the form 6n-1 and 6n+1. A pair of twin primes are formed by 6(215)+1 and 6(215)-1
A nice foot note to this fact is that the 215th and 216 primes are twin primes.
215 is the sum of discrete factorials, 8! + 7! + 6! + 5! + 4! + 1!.
215 is the difference of two cubes, 6^3 - 1^3.
24 arrangements of (2,3,7,42), (2,3,8,24), (2,3,9,18), (2,3,10,15), (2,4,5,20) and (2,4,6,12).
12 arrangements of (3,3,4,12), (3,4,4,6), (2,3,12,12), (2,4,8,8) and (2,5,5,10).
6 arrangements of (3,3,6,6).
4 arrangements of (2,6,6,6).
1 arrangement of (4,4,4,4).
The 216th Day of the Year
216= 6^3 = 2^3 + 3^3; I'm calling such numbers cube-full, in analogy to square-full numbers, (see 196) since every prime p that divides 216,p^2 and p^3 will also divide 216. Only one more year day is a perfect cube.
According to *Derek Orr, m^k is the largest number n such that (n^k-m)/(n-m) is an integer (for k > 1 and m > 1). So no number n larger than 216 will make (n^3 - 6 )/(n-6) is an integer. When n = 216, the quotient is 47989 (Hope I got that right).
Because 216/4=54, it must be the difference of two squares, 55^2-53^2 , and because 216/8 = 27, 216 = 29^2 - 25^2
Also, because 8*25 + 16 = 216, it is the difference of two squares of the numbers form n and n+4; in this case 29^2 - 25^2=216. (two different rules that gave the same solution. and because 216 = 12 * 18, 216 = 21^2 - 15^2
Since 216 = 33 + 43 + 53 = 63, it is the smallest cube that's also the sum of three cubes (Plato was among the first to notice this, and mentioned it in Book VIII of Republic). (What is the next cube that is the sum of three cubes?... and how can you be sure there will never be a day that is a cube that is the sum of two cubes?)
According to the Ken Burns series Baseball, 216 is the number of stitches on a baseball.*Wikipedia
Zhi-Wei Sun conjectured in March 2008 that 216 is the only number not of the form p + k(k+1)/2, with p = 0 or p prime. *Prime Curios
6 of one and a dozen and a half of another, The numbers 6*216+1, 12*216+1, and 18*216+1 are all prime *Prime Curios
6^3 = 216 = 31 + 33 + 35 + 37 + 39 + 41 (OK so what?) but if
5^3 = 125 = 21 + 23 + 25 + 27 + 29 and
4^3 = 64 = 13 + 15 + 17 + 19 and
3^3 = 27 = 7 + 9 + 11 and
2^3 = 8 = 3 + 5 and you know
1^3 = 1 = 1 , you might wonder if 7^3 = 343 might be...
216 is a balanced binary number, having four ones, and four zeros.
216 is a palindrome in base five, (1331) =5^3 + 3*5^2 + 3 * 5 + 1 =216
216 is also the sum of a twin prime pair (107 + 109).
A multiplicative Magic Square with a constant of 216.
2 9 12
36 6 1
3 4 18
*Wikipedia
The smallest and only known cube sandwiched between two triplets of semiprimes (i.e., 213=3*71, 214=2*107, 215=5*43 and 217=7*31, 218=2*109, 219=3*73). *Prime Curios
There are 216 fixed hexominoes, the polyominoes made from 6 squares. *Wikipedia
And for those who are interested in variations on 666, the so called number of the beast, \( 6^{(6^6)} =216 \) mod 360 from which we get the divine equivalent, \( cos(216)^o= \frac{- \phi}{2} \)
Ok, one more 216 to 666 connection from Ben Vitalle. There is a right triangle with legs of 216, 630 and a hypotenuse of (wait for it...... ) 666 Benjamin Vitale @BenVitale
216 is the smallest number that may be expressed as the difference of the sums of the squares of successive twin primes (13^2 + 11^2) - (7^2 + 5^2).*Prime Curos
216 is the third year day for which the number plus the sum of the cubes of its digits is a square, 216 + 2^3 + 1^3 + 6^3 = 21^2
The 217th Day of the Year
217 is both the sum of two positive cubes and the difference of two positive consecutive cubes in exactly one way: 217 = 63 + 13 = 93 − 83. (How frequently would the difference of two consecutive cubes also be expressible as the sum of two cubes?)
217 is the sum of four cubes, 1^3 + 3^3 + 4^3 + 5^3
217 is a palindrome in base six, (1001) and in base 12, (161).
Anti-sigma(n) is not a well known function, but anti-sigma(22) = 217. Simply add all the numbers from one to 22, then subtract all the numbers that divide into 22 evenly: [ (22*23)/2 - 1 - 2 - 11 - 22 = 217 ]
217 = 109^2-108^2 = 19^2-12^2
217^2 = 47089. Both 47 and 89 are primes, as is their concatenation, 4789. *Prime Curios
217 = 7 x 31, the first of three consecutive semi-primes.
A 7x7 Magic square with integers 7 - 53 has a magic sum of 217 *@SrinivasR1729
217 is the ninth centered hexagonal number.
The 218th Day of the Year
109 is the sum of two squares, 10^2 + 3^2. Can you see how to use this to get the sum of squares of 2x 109?
218 = 72 + 132
218 = 6^3 + 1^3 + 1^3, and the difference of two cubes, 7^3 - 5^3.
218 is the number of nonequivalent ways to color the 12 edges of a cube using at most 2 colors, where two colorings are equivalent if they differ only by a rotation of the cube.
The sum of its digits is 11, the sum of its prime factors is 111.
The 217th Day of the Year
217 is both the sum of two positive cubes and the difference of two positive consecutive cubes in exactly one way: 217 = 63 + 13 = 93 − 83. (How frequently would the difference of two consecutive cubes also be expressible as the sum of two cubes?)
217 is the sum of four cubes, 1^3 + 3^3 + 4^3 + 5^3
217 is a palindrome in base six, (1001) and in base 12, (161).
Anti-sigma(n) is not a well known function, but anti-sigma(22) = 217. Simply add all the numbers from one to 22, then subtract all the numbers that divide into 22 evenly: [ (22*23)/2 - 1 - 2 - 11 - 22 = 217 ]
217 = 109^2-108^2 = 19^2-12^2
217^2 = 47089. Both 47 and 89 are primes, as is their concatenation, 4789. *Prime Curios
217 = 7 x 31, the first of three consecutive semi-primes.
A 7x7 Magic square with integers 7 - 53 has a magic sum of 217 *@SrinivasR1729
217 is the ninth centered hexagonal number.
 |
| OEIS |
The 218th Day of the Year
109 is the sum of two squares, 10^2 + 3^2. Can you see how to use this to get the sum of squares of 2x 109?
218 = 72 + 132
218 = 6^3 + 1^3 + 1^3, and the difference of two cubes, 7^3 - 5^3.
218 is the number of nonequivalent ways to color the 12 edges of a cube using at most 2 colors, where two colorings are equivalent if they differ only by a rotation of the cube.
The sum of its digits is 11, the sum of its prime factors is 111.
218 is a palindrome in base 9, 262_9
218 is the smallest number with a Merten funtion =3. (an acceptable definition for students is that the Merten number for n, M(n), is the count of square-free integers up to n that have an even number of prime factors, minus the count of those that have an odd number.) The function is named in honor of Franz Merten, who was a teacher of Schrodinger.
218 is the number of points on a 6x6x6 space lattice
The 219th Day of the Year
219 is an odd number, so it is the difference of two consecutive squares, 219 = 110^2 - 109^2, and because 219 = 6n+9 for n=35, then 219 = 38^2 - 25^2
The Merten Function of 219 (see 218) = 4, a second day hitting a record high.
There are 219 space groups in 3 dimensions, analogous to the 17 wallpaper groups in 2 dimensions.
219 p +2 is prime when p is any of the first three primes. Srinivase Raghava adds that it is also true with exponents of 6, 23, 34, 35, 36, and 64.
219 is a palindrome in binary, (11011011), and a repdigit in base 8 (333). And I think it is kind of cute that in base 36, it is (63)
219 is the sum of four cubes (not all distinct) and in more than one way. One way is 6^3 + 1^3 + 1^3 + 1^3, can you find the other?
There are 219 ways to partition 37 into prime parts.
219 is a repdigit in base 8 (octal) 333_8
219 is a Happy Number, the iteration of the sum of the squares of the digits eventually maps to one.
The 220th Day of the Year
220 is the smallest amicable number, paired with 284. Amicable numbers are two different numbers so related that the sum of the proper divisors of each is equal to the other. Amicable numbers were known to the Pythagoreans, who credited them with many mystical properties. (what is the next pair?)
If you add the sum of all the divisors of the first 16 numbers, you get 220.
220 is the the largest difference between two consecutive primes less than 100,000,000.
220 is the tenth tetrahedral number, the sum of the first ten triangular numbers, 1 + 3 + 6 + ... + 55 *Wikipedia
220 is a pseudo-perfect number, the sum of a subset of its proper divisors. 220 = 110 + 55 + 44 + 11. Almost all abundant numbers (the sum of its proper divisors is greater than the number) are pseudoperfect, but Not quite all. Can you find the smallest of these 'weird" abundant numbers that are not pseudoperfect.
220 is a tetrahedral number, the sum of the first ten triangular numbers. 220= 1 + 3 + 6 + ...+ 55
Finding a number whose reciprocal is equal to the sum of two other reciprocals is an important idea in many areas of math and science. 220 turns out to be the largest of a triple of that type, 1/220 + 1/180 = 1/99
Because 220/4 = 55, 220 = 56^2 - 54^2,
If all the diagonals of a regular dodecagon are drawn, they divide the dodecagon into 220 regions.
220 is the sum of four consecutive primes. 47 + 53 + 59 + 61.
Interesting that if you multiply the first 220 composite numbers, it is a composite number that falls between a pair of twin primes. *Prime Curios
Every number less than 220 can be formed by the sum of divisors of 220 .
220 is the largest gap between consecutive primes less than 10^8.
And Derek Orr Points out that the aliquot(proper divisors) sequence for 220 does not end in 1. This was known to the ancients who described such numbers as amicable numbers. 220 and 228 are each the aliquote sum of the other. There are a few other numbers which have repeating sequences of three or more in a loop. The aliquot sequence of perfect numbers is fixed by their definition. There are other non-perfect numbers whose sequence eventually lands on a perfect number and then repeats that number infinitly (are until you stop calculating). and there are a few other numbers which have repeating sequences of three or more in a loop.
You can make a 5x5 magic square with consecutive numbers from 32 to 44, following the order of the standard 5x5 magic square.... or simply add 31 to each one in the primitive square.
The 221st Day of the Year
221 represents the hypotenuse of four Pythagoren triangles, (21^2+220^2=221^2), (85^2+204^2=221^2), (104^2+195^2=221^2), (140^2+171^2=221^2). 221 is also expressible as the sum of two squares in two different ways: (5^2+14^2 = 221 = 10^2+11^2). *Prime Curios
If you deal two cards at random from a standard deck, your chances of getting two aces, is 1 in 221.
221 the sum of consecutive prime numbers in two different ways 221 = (37 + 41 + 43 + 47 + 53) = (11 + 13 + 17 + 19 + 23 + 29 + 31 + 37 + 41)
And of course, 221 is the product of consecutive primes, 13 x 17
Because 13 and 17 are both 4n+1 primes, they are the sum of two squares (13= 3^2 + 2^2and 17 = 4^2 + 1^2), these can be used to construct two ways that 221 can be shown as the sum of two primes. (221 = (3*4+1x2)^2 + (4x2-1x3)^2 = 14^2 + 5^2 and by changing +/- (3x4-1x2)^2 + (4x2+1+3)^2 = 10^2 X 11^2 = 221. Known around 1st century AD by Diophontas.
221221 + 122 is prime, it is the only known number greater than one with this property.
221 = 111^2 - 110^2. (All odd numbers are the difference of consecutive squares.)
220 is the sum of four consecutive primes. 47 + 53 + 59 + 61.
Interesting that if you multiply the first 220 composite numbers, it is a composite number that falls between a pair of twin primes. *Prime Curios
Every number less than 220 can be formed by the sum of divisors of 220 .
220 is the largest gap between consecutive primes less than 10^8.
And Derek Orr Points out that the aliquot(proper divisors) sequence for 220 does not end in 1. This was known to the ancients who described such numbers as amicable numbers. 220 and 228 are each the aliquote sum of the other. There are a few other numbers which have repeating sequences of three or more in a loop. The aliquot sequence of perfect numbers is fixed by their definition. There are other non-perfect numbers whose sequence eventually lands on a perfect number and then repeats that number infinitly (are until you stop calculating). and there are a few other numbers which have repeating sequences of three or more in a loop.
You can make a 5x5 magic square with consecutive numbers from 32 to 44, following the order of the standard 5x5 magic square.... or simply add 31 to each one in the primitive square.
The 221st Day of the Year
221 represents the hypotenuse of four Pythagoren triangles, (21^2+220^2=221^2), (85^2+204^2=221^2), (104^2+195^2=221^2), (140^2+171^2=221^2). 221 is also expressible as the sum of two squares in two different ways: (5^2+14^2 = 221 = 10^2+11^2). *Prime Curios
If you deal two cards at random from a standard deck, your chances of getting two aces, is 1 in 221.
221 the sum of consecutive prime numbers in two different ways 221 = (37 + 41 + 43 + 47 + 53) = (11 + 13 + 17 + 19 + 23 + 29 + 31 + 37 + 41)
And of course, 221 is the product of consecutive primes, 13 x 17
Because 13 and 17 are both 4n+1 primes, they are the sum of two squares (13= 3^2 + 2^2and 17 = 4^2 + 1^2), these can be used to construct two ways that 221 can be shown as the sum of two primes. (221 = (3*4+1x2)^2 + (4x2-1x3)^2 = 14^2 + 5^2 and by changing +/- (3x4-1x2)^2 + (4x2+1+3)^2 = 10^2 X 11^2 = 221. Known around 1st century AD by Diophontas.
221221 + 122 is prime, it is the only known number greater than one with this property.
221 = 111^2 - 110^2. (All odd numbers are the difference of consecutive squares.)
221 is an arithmetic number, the mean of its divisors is a whole number; (1 + 13 + 17 + 221) /4 = 63
221 is the number of 7-vertex Hamiltonian planar graphs ( a graph that allows a closed path that visits each node exactly once.)
2*3*5*7+11=13*17. Note the consecutive use of the first 7 primes. *Prime Curios
221 in hexdecimal (base 16) is given by (DD) or (13, 13) 13*16 + 13.
221 is the number of 7-vertex Hamiltonian planar graphs ( a graph that allows a closed path that visits each node exactly once.)
2*3*5*7+11=13*17. Note the consecutive use of the first 7 primes. *Prime Curios
221 in hexdecimal (base 16) is given by (DD) or (13, 13) 13*16 + 13.
221 is also a palindrome in base 7 (434) and base 11 (191).
The 222nd Day of the Year
A repdigit, 222 = 2 x 3 x 37
222 is called a sphenic (Greek for wedge) number. They have three distinct prime factors. 30= 2x3x5 is the smallest sphenic number. Can you find two consecutive numbers that are both sphenic numbers? More??
481 / 222 = (4+8+1)/(2+2+2) = 13/6 *Potetoichiro
222 = (3!)3 + (2!)2 + (1!)1 + (0!)0 *Derek Orr @Derektionary
222 is the number of lattices on 10 unlabeled nodes.
and 222 is the sum of consecutive primes. 109 + 113, No larger year date shares this quality
222 is the smallest repdigit such that the product of itself and all truncations of itself plus and minus one results in twin primes. I.e., 222*22*2 ± 1 are twin primes. *Prime Curios
The digit sum of 222 in base ten is the same as digit sum in binary, and also in base three.
222 is the sum of all two digit primes formed by consecutive digits. *Prime Curios
222 is also a repdigit in base 36 (66)
222 is called a pseudo-perfect number, it is the sum of a subset of its divisors, 37+74 + 111 = 222
The 223rd day of the Year
223 is the 48th prime number, formed from three consective prime digits, and the sum of three consecutive primes (71 + 73 + 79), 223 and also the sum of seven consecutive primes (19 + 23 + 29 + 31 + 37 + 41 + 43)
Every number can be formed with no more than 36 fifth powers, except one, 223 is the only number that requires 37 fifth powers. This is related to Waring'a problem. In number theory, Waring's problem asks whether each natural number k has an associated positive integer s such that every natural number is the sum of at most s natural numbers to the power of k. For example, every natural number is the sum of at most 4 squares, 9 cubes, or 19 fourth powers. Waring's problem was proposed in 1770 by Edward Waring, after whom it is named. Its affirmative answer, known as the Hilbert–Waring theorem, was provided by Hilbert in 1909.
Fans of Star Wars may know that this is sometimes called the Star Wars Droid Prime because it uses only the numbers in the names of R2-D2 and C3PO
An interesting note, if you take a prime number less than 223 and reverse it, some are prime, some have two factors, but 223 is the smallest prime whose reversal has three factors (322 = 2 * 7 * 23)
223 is the difference of consecutive squares, 112^2 - 111^2
The number of primes and the number of composites that cannot be written as the sum of two primes, up to 223, are equal.*Prime Curios
The prime preceding 223 The sums of the nth powers of its digits are prime for all n between 1 and 6 inclusive: sum of digits = 7, sum of squares of digits = 17, sum of cubes of digits = 43, sum of fourth powers = 113, sum of fifth powers = 307 and sum of sixth powers = 857. *Prime Curios
If you take the tens compliment of the digits of 223, you get 887, another prime. The same is true for the next three primes following 223.
If you take the square of 223 which is 49729 observe that the last three digits, 729 are prime. Try any of the next dozen primes following 223 and you will observe the same result. *Prime Curios
223 sets a new high for the distance between the two nearest primes surrounding it, they are 16 units apart. *Wikipedia
The 224th Day of the Year
224 is the sum of the cubes of 4 consecutive integers:
224 = 23 + 33 + 43 + 53 and also 23 + 63
Cool thing about 224: 224 = 23+45+67+89 *Derek Orr @Derektionary.
Every number smaller than 224 can be expressed as the sum of distinct divisors of 224.
224 = 2^5 x 7. With so many factors of two, it has lots of representations as the difference of two squares. 224 = 57^2 - 55^2 = 30^2 - 26^2 = 15^2 - 1^2
224 is a palindrome in base 3 (22022).
14 red checkers and 14 black checkers on a string of 15 squares can be exchanged by sliding, or jumping in 224 moves.
224 is a Harshad number (Joy-giver), divisible by the sum of its digits. It is also divisible by the product of its digits. Of all the year days that meet both conditions, only two are not divisible by three. 224 is the larger of the two.
224 is one more than a prime, and one less than a square, and its square, 50176, is one less than a prime.
The 225th Day of the Year
225 = 01 + 23 + 45 + 67 + 89 *HT to Derek Orr
225 is the smallest square that can be can be written as the sum of a cube and a square (a^3 + b^2) in two ways, i.e., 225=5^3+10^2=6^3+3^2*Prime Curios
I'm still pushing for the symbol \(n!_2 \); for (n * (n-2) * ... what some call the double factorial. So \(225 = (5!_2)^2 =(5 * 3 * 1)^2\)
Also (4^2 - 1) ^2
224 is one more than a prime, and one less than a square, and its square, 50176, is one less than a prime.
The 225th Day of the Year
225 = 01 + 23 + 45 + 67 + 89 *HT to Derek Orr
Playing around with numbers n, such that n^k has a digit sum of n, turns out that 225^21 = 24878997722115027320114677422679960727691650390625, and the digit sum, 225.
225 is the ONLY three digit square with all prime digits. Can you find a four digit square with all prime digits?
225 = (3!)3+(2!)3+ (1!)3
\(225 = 1^3 + 2^3 + 3^3 + 4^3 + 5^3 \) (which means, of course, that \( 225 = (1+2+3+4+5)^2 \) *Derek Orr @Derektionary It is the last year day that is the sum of five consecutive cubes.
225^n + 2 is prime for half the values of n from 1 to 10. *Prime Curios
225 is the smallest number that is a polygonal number in five different ways. It is a square number (225 = 15^2), an octagonal number, and a squared triangular number (225 =15^2 = (1 + 2 + 3 + 4 + 5)^2 = 1^3 + 2^3 + 3^3 + 4^3 + 5^3) (Student note. The square of the nth triangular number is always the sum of the first n cubes. For example 1^2 + 2^2 + 3^3 = (1+2+4=3)^2 = 36. This identity is sometimes called Nicomachus's theorem, after Nicomachus of Gerasa (c. 60 – c. 120 CE). 225 is the last year day that is the sum of the first n cubes. *Wikipedia
225 is the ONLY three digit square with all prime digits. Can you find a four digit square with all prime digits?
225 = (3!)3+(2!)3+ (1!)3
\(225 = 1^3 + 2^3 + 3^3 + 4^3 + 5^3 \) (which means, of course, that \( 225 = (1+2+3+4+5)^2 \) *Derek Orr @Derektionary It is the last year day that is the sum of five consecutive cubes.
225^n + 2 is prime for half the values of n from 1 to 10. *Prime Curios
225 is the smallest number that is a polygonal number in five different ways. It is a square number (225 = 15^2), an octagonal number, and a squared triangular number (225 =15^2 = (1 + 2 + 3 + 4 + 5)^2 = 1^3 + 2^3 + 3^3 + 4^3 + 5^3) (Student note. The square of the nth triangular number is always the sum of the first n cubes. For example 1^2 + 2^2 + 3^3 = (1+2+4=3)^2 = 36. This identity is sometimes called Nicomachus's theorem, after Nicomachus of Gerasa (c. 60 – c. 120 CE). 225 is the last year day that is the sum of the first n cubes. *Wikipedia
225 is the smallest square that can be can be written as the sum of a cube and a square (a^3 + b^2) in two ways, i.e., 225=5^3+10^2=6^3+3^2*Prime Curios
I'm still pushing for the symbol \(n!_2 \); for (n * (n-2) * ... what some call the double factorial. So \(225 = (5!_2)^2 =(5 * 3 * 1)^2\)
Also (4^2 - 1) ^2
If you take the normal 3x3 magic square, and multiply each digit by 15, you get a magic square with a constant of 225. Or just add 70 to each value and get a different one using 71-79 with 225 for the magic constant
\(225 = 15^2 , and 225 = 25^2 - 20^2 = 113^2-112^2 = 39^2 - 36^2 \)
225 is a centered Octagonal number, here they are 1, 9, 25, 49, 81, 121, 169, 225, 289, 361, 441, 529, ... notice they are the odd squares. Also of interest because the sum of their reciprocals is \(\frac{\pi^2}{8}\)
The 226th Day of the Year
The iteration of the sum of the squares of the digits leads to one (a happy number). What percentage of numbers have this property?
226 = 3!3+2!3+1!3 + 0!3 *Derek Orr
The binary expression for 226 has the same number of ones and zeros. There are only 49 such year days, and this is number 46.
226 is one more than a square, and one less than a prime. (Numbers one more than a power, 226=15^2+1 for example, are called Cunningham numbers after English mathematician A. J. C. Cunningham (1842 - 1928).
226 =15^2 + 1^2. So what might you learn from seeing that written as (8+7)^2 + (8-7)^2?
226 has a totally balanced binary expression, with four ones and four zeros.
226 is the tenth centered pentagonal number.
The 227th Day of the Year
227 is a prime number, and the smaller of a twin prime pair; but it can also be written as the sum of the sum and the product of the first four primes: (2 + 3 + 5 + 7)+(2 x 3 x 5 x 7) = 227.
In a similar way, the first two primes work (2+3)+(2x3)=11 is prime. Can you find another? (Ben Vitale has found all the cases under 1000 for which p = (a + b + c + … ) + (a * b * c …) He even found another way to express 227. His blog also has lots of other number curiosities, so give it a look. Much fun.
227 is also the largest odd day number of the year which can NOT be expressed as a prime added to twice a square. There are three others you might fine, and three others larger than 366. Observe that these are all seven prime.[OEIS gives ten numbers that include 1, 5779, and 5993. These last two are composite. and that seems to be ALL of them that exist. if there are more, they are larger than 10^13.)
This problem is based on an original conjecture by C Goldbach that all ODD COMPOSITE numbers could be written as twice a square plus a prime. The laxt two show he was wrong.
227 is the 7^2 prime number *Prime Curios
The harmonic sequence, or sum of the reciprocals of the integers grows to infinity, but slowly. It takes the 227th term (1/227) to finally push it over the value 6.(And don't even think about trying to get to seven!)
A beauty about six primes, 227 + 251 + 257 = 233 + 239 + 263, and if you square each one, 227^2 + 251^2 + 257^2 = 233^2 + 239^2 + 263^2 *Prime Curios.
227 is a palindrome in base eight (343)
The number (7 * 10^227+71)/3 Forms a prime with the digit 2 followed by 225 digits of 3, then ending in 57
Students might try a few starting with 3, ,4, etc.
22/7 is a common approximation for Pi in middle school.
There are 227 composite days in a year. *Prime Curios
The 228th Day of the Year
228 is the number of ways, up to rotation and reflection, of dissecting a regular 11-gon into 9 triangles.
228 + 1, 822 + 1, and (228 + 822) + 1 are all primes. *Prime Curios Is there another such year day?
228 in binary is written 11100100 notice that this is all four possible two digit binary combinations in descending order, 11, 10, 01, 00. (Just figured out that the equivalent in base three is a little over 1039)
228/4 = 57 so 228 = 58^2 - 56^2.
228 is a palindrome repdigit in base 7, (444)
228 is a palindrome in base 12 (171). (I resist using "duodecimal system" because I once mis-spoke in class and said "Dewey decimal system" twice in one brief comment. My students that year seemed to have thousands of comments about trivia related to the actual Dewey Decimal system that they would share with each other in class.)
228 is divisible by the sum of its digits, 12, and the number of divisors it has, also 12. How frequent is this?
If the sum of its digits,12, is subtracted from 228, you get a cube, 228 - 12 = 216 = 6^3. If the product of its digits, 32, is subtracted, you get a square. 228 - 32 = 196 = 14^2
The 229th Day of the Year
229 is the 50th prime, and is the smallest prime that added up to the reversal of its digits yields another prime, (229 + 922) = 1151 (can you find the next one?)
The sum of the digits of 229 is prime (13) and the sum of squares of the digits is also prime (89).
It can be written as a sum of positive squares in only one way, i.e., 225 + 4 = 15^2 + 2^2 .
The 229th Day of the Year
229 is the 50th prime, and is the smallest prime that added up to the reversal of its digits yields another prime, (229 + 922) = 1151 (can you find the next one?)
The sum of the digits of 229 is prime (13) and the sum of squares of the digits is also prime (89).
It can be written as a sum of positive squares in only one way, i.e., 225 + 4 = 15^2 + 2^2 .
extra: 229 is the difference between 3³ and 4⁴ *jim wilder @wilderlab
If you replace each digit of 229 with its square, you get a prime number, 4481. If you do the same with its cube, you get 88729, another prime. *Prime Curios
1/229 has 228 digits in its period
If you replace each digit with its ten complement, you get 881, another prime.
100! + 229 is prime
2^229 is a 69-digit number containing only one zero. Is this the largest power of two that has one or more unique digits? *Prime Curios
If you draw K13, the complete graph with 13 vertices so that it has the fewest possible crossings, it will still have 229 crossings *Wikipedia
1/229 has 228 digits in its period
The 230th Day of the Year
230 is the smallest number such that it and the next number are both sphenic numbers, the product of three distinct primes (230 = 2*5*23 and 231 = 3*7*11). *Prime Curios (can there be three consecutive numbers that are the product of three (or n) distinct primes?
Their are 230 possible crystal shapes that can tile space (this counts the chiral reflections as separate). This is the analogy of the better known 17 "wallpaper groups" which tile the plane. Interestingly, both were proved by the same man, Evgraf Fedorov, in 1891. He did the plane problem the hard way, he proved the case for space first, then worked backwards to the plane.
230 is a Harshad number, divisible by the sum of its digits
And 230 is another Happy number.
The sum of the proper divisors of 230 is 202. Iterating this process on each new number gives 104, 106, 56, 64, 63, 41, 1, 0,
230^2 + 1 = 52901, a prime
230 is the sum of four consecutive Triangular numbers; 21 + 28 + 36 + 45. Also the sum of four consecutive squares; 36 + 49 + 64 + 81
The 231st Day of the Year
there are 231 cubic inches in a US Gallon, (admit it, you did NOT know that.)
Ok, and it's also the sum of the squares of four distinct primes, 231 = 22 + 32 + 72 + 132.
\((3!)^3 + (2!)^4 - (1!)^5 \)
231 = 12 + 23 + 34 + 45 + 56 + 61 (loop 1-2-3-4-5-6-1)
231 = 98 + 76 + 54 + 3
*Derek Orr
The prime factorization of 231 is 3 x 7 x 11, three prines in arithmetic progression. There are only two year days with this quality, and 231 is the larger. It was not until 1933 that a proof was found that there are infinitely many such arithmetic progressions.
The 231st Day of the Year
there are 231 cubic inches in a US Gallon, (admit it, you did NOT know that.)
Ok, and it's also the sum of the squares of four distinct primes, 231 = 22 + 32 + 72 + 132.
\((3!)^3 + (2!)^4 - (1!)^5 \)
231 = 12 + 23 + 34 + 45 + 56 + 61 (loop 1-2-3-4-5-6-1)
231 = 98 + 76 + 54 + 3
*Derek Orr
The prime factorization of 231 is 3 x 7 x 11, three prines in arithmetic progression. There are only two year days with this quality, and 231 is the larger. It was not until 1933 that a proof was found that there are infinitely many such arithmetic progressions.
231 = 40^2-37^2 = 20^2 - 13^2
231 in base twenty seems as small as a BB. (11 x 20 + 11)
The average number of distinct prime divisors for all n less than a googolplex is only about 231. *Prime Curios
The sum of the first 21 integers, 231 is the 21st triangular number, It is also a hexagonal and an octahedral number.
 |
| *Wikipedia |
Their are 231 integer partitions of 16.
Here are the seven partitions of 231 into strings of consecutive counting numbers
231=1+2+3+4+5+6+7+8+9+10+11+12+13+14+15+16+17+18+19+20+21
231=10+11+12+13+14+15+16+17+18+19+20+21+22+23
231=16+17+18+19+20+21+22+23+24+25+26
231=30+31+32+33+34+35+36
231=36+37+38+39+40+41
231=76+77+78
231 =115+116
*Hansreudi Widmer
The 232nd Day of the Year
232 is the maximum number of regions that the plane can be divided into with 21 lines (how many of the regions would be of infinite area?)
232 is a palindrome in base ten, but no other base from 2-9
There are 232 bracelets possible with 8 beads of one color and seven of another.
And from Derek Orr
232 is sum of the cubes of the factorials of its digits,
232 = (2!)3 + (3!)3 + (2!)3
and 232 the sum of the first 11 Fibonacci numbers. 232 = 1+1+2+3+5+8+13+21+34+55+89
If you add up all the proper divisors of a number, n, they can be less than n, as 231 is(deficient), equal to n (perfect, like 6 or 28) or abundant. 12 is the smallest abundant number. Nicomachus wrote only of even numbers because he thought all odd numbers were deficient, but he was wrong. The 232nd abundant number is odd, 945.
If you raise 232 to the power of the product of its digits, and then add the sum of its digits, you get a prime. The only other known number with this property is 187. *Prime Curios
232 /4 = 58, so 59^2 - 57^2 = 232. 232 / 8 = 29 so 31^2-27^2 = 232.
Because 58 is a sum of two squares, 232 is also. 58 =7^2 + 3^2, 232 = 14^2 + 6^2. Students might use this to find the sum of two squares for 522 = 9 x 58.
232 is another balanced binary, with four of each ones and zeros, and always the number of ones is greater than, or equal to, the number of zeros.
232 is one less than a Fibonacci Prime.
The 233rd Day of the Year
233 is the only three digit prime that is also a Fibonacci number. It is the only known Fibonacci prime whose digits are all Fibonacci primes, and the sum of its digits is also a Fibonacci number. *Prime Curios
233 is also the last day of the year that is the sum of the squares of consecutive Fibonacci numbers. (A pretty mathematical fact for the day: the sum of the squares of two consecutive Fibonacci numbers is always a Fibonacci number, Students can show that the converse is not true.) If you square 8 (F6) and add the square of 13(F7) you get 233 (F(6+7))
There are exactly 233 maximal planar graphs with ten vertices, and 233 connected topological spaces with four points.
The ratio of 233/144, two Fibonacci numbers is a good approximation to Phi, the Golden Ratio.
233 is the smallest prime factor of |(2^{29} - 1 \) *Prime Curios
If you start the "see and say" sequence with 233 (a prime), you get 1223, another prime. And if you do it again with 1233, you get another. *Prime Curios
233 is the smallest magic constant of a 5x5 prime Magic square. It uses all the odd primes below 100. *Prime Curios
233 is the sum of the squares of the first four semi-primes, 4^2 + 6^2 + 9^2 + 10^2.
233= 117^2 - 116^2
233 is the sum of eleven consecutive primes, 5 + 7 + 11 + ... + 41
233 is a palindrome in base 3 (22122)
233 in Roman numerals uses 2 C's, 3 X's, and 3 I's. One of the Roman Numeral displays that you can find digital root by counting the number of symbols. Any number using only M, C, X and I will also work
The 234th Day of the Year
234 begins a string of eight consecutive digits which is a prime number, 23456789. All the children need to know why there can not be any ordering of all nine digits , 1 to 9 that is prime. Teach one today. Casting out nines is as old as Iamblichus, and as new as the youngest kid entering kindegarten.
234 is the 55th abundant number. It's proper divisors are {1, 2, 3, 6, 9, 13, 18, 26, 39, 78, 117} and their sum is greater than 234, it is 312. There are 86 abundant numbers in a non-leap year. 234=6(39) and all multiples two or greater of a perfect number are abundant.
9^2 + 6^2 = 117. Can you see how that tells you that 234 = 3^2 + 15^2???
234 is the number of ways to partition 50 intoat most three parts.
The 235th Day of the Year
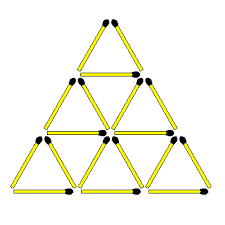
235 is the number of trees with 11 vertices. (Counting the number of unlabeled free trees is still an open problem in math. No closed formula for the number of trees with n vertices up to graph isomorphism is known.)
If you build an equilateral triangle with nine matchsticks on each side, then subdivide into additional equilateral triangles, there will be a total of 235 triangles of several different sizes. The image shows the subdivision of a equilateral triangle with three matchsticks on a side. Can you find the thirteen triangles in it?
235 is a semi-prime (5 x 47) that is the concatenation of the first three Fibonacci primes
235 is a palindrome in base 4 (32234), 7 (4547), and 8 (3538),
U-235 is the fissile isotope of uranium used in the first atomic bombs.
235, like all numbers ending in 5 that are greater than 25, is the difference of two squares of integers that differ by five, 26^2 - 21^2 = 235. Like all odd numbers, it is the difference of two consecutive squares, 235 = 118^2 - 117^2.
235 is the tenth Heptagonal (7-gon) number. n*(5n-3)/2 where n =10
The 236th Day of the Year
236 is the sum of twelve consecutive primes, 3 + 5 + 7 + 11 + 13 + 17 + 19 + 23 + 29 + 31 + 37 + 41
And 236 is the number of possible positions in Othello after 2 moves by both players. *Erich Friedman (Students might try to figure out how many possible positions are there in tic-tac-toe after 2 moves by each player. Entice them with this number fact from Cliff Pickover @pickover )
The sum of the divisors of 236, 2+2 + 59 =63, the product of it's digits is 2x3x6 = 36, the reversal of the sum of the divisors.*Prime Curios
236^2 + 1 is a prime
236 is the average of two consecutive primes 233 and 239.
236 is a Happy Number. The sum of the squares of the digits under iteration go to 1.
Since 236/4 = 59, 236 = 60^2 - 58^2.
The 237th Day of the Year
it would be a singularly uninteresting number (3 x 79) except that the room number in the film, "The Shining" was switched from 217 in the novel to 237 for the film? It seems that the Timberline Lodge had a room 217 but no room 237, so the hotel management asked Kubrick to change the room number because they were afraid their guests might not want to stay in room 217 after seeing the film. *Visual Memory.co.uk
Derek Orr added, 237 = 44th prime + 44 = 193 + 44 What's the next number that equals the n-th prime + n?
237 is the difference of two consecutive squares, like all odd integers, 119^2 - 118^2 = 237, but also 41^2 - 38^2 because 6*38+9 = 237.
The 237th square pyramidal number, 4465475, is also a sum of two smaller square pyramidal numbers. There are only four smaller numbers (55, 70, 147, and 226) with the same property. *Wikipedia
237 is a lucky number, it remains after all sieves in the Eratosthenes-like Sieve of Stan Ulam. " Start with the natural numbers. Delete every 2nd number, leaving 1 3 5 7 ...; the 2nd number remaining is 3, so delete every 3rd number, leaving 1 3 7 9 13 15 ...; now delete every 7th number, leaving 1 3 7 9 13 ...; now delete every 9th number; etc." *OEIS
237 is the last of three consecutive odd numbers that have all prime digits.
237 not only has all prime digits, any substring of 2 consecutive digits also form a prime, 23, 37.
The 238th Day of the Year
The 238th day of the year; 238 is an untouchable number, The untouchable numbers are those that are not the sum of the proper divisors of any number. 2 and 5 are untouchable, can you find the next one? (four is not untouchable, for example since 1+3=4 and they are the proper divisors of 9) Five is the only known odd untouchable number.
238 is also the sum of the first 13 primes, and its digits add up to ........wait for it.... 13 (2+3+8 = 13 and 238 = sum of first 13 primes). Only two more year dates is the sum of the first n primes.
23=8 (We are tentatively calling these "power equation numbers") *Derek Orr
In base 16 238 is a Repdigit, EE (14 x 16 + 14
238 is the number of partitions of 34 into powers of two.And also the same for 35.
6 times 238 is between a pair of twin primes.
The 239th Day of the Year
When expressing 239 as a sum of square numbers, 4 squares are required, which is the maximum that any integer can require; it is the largest number that needs the maximum number (9) of positive cubes. 239 = 5^3 + 3^3 + 3^3 + 3^3 + 2^3 + 2^3 + 2^3 + 2^3 + 1^3. (Only one other number requires nine cubes, can you find it?)
and a hundred years (+/-) ago (many people included 1 as a prime then; see more) 239 would have been a prime that is the sum of the first 14 primes; 239 = 1+2+3+5+7+11+...+37+41 *Derek Orr
The sum of the odd numbers from 1 to 239 is equal to the sum of the odd numbers from 239 to 337.
239 appears in one of the earliest known geometrically converging formulas for computing Pi: Pi/4 = 4 arctan(1/5) - arctan(1/239) *.archimedes-lab.org
239 is the 52nd prime, and the smaller of a pair of twin primes with 241.
For any number, greater than 239, the largest factor of n^2 + 1 is at least 17. *Prime Curios
If you factor 1234567654321, the smallest prime factor is 239. *Prime Curios
Arctan(1/239) in degrees begins 0.239..., and this is the only positive integer for which this is true. *Prime Curios
The 52nd prime number (239) has a Collatz trajectory length of 52. It is also the smallest integer greater than 1 that has the same Collatz trajectory length as its square (57121) *Prime Cuiros
There are 239 primes less than 1500.
239/169 is a convergent of the continued fraction of the square root of 2, so is is a solution to the Pell Equation 239^2 = 2 · 169^2 − 1.
The only solutions of the Diophantine equation y^2 + 1 = 2x^4 in positive integers are (x, y) = (1, 1) or (13, 239).*Wikipedia
The 240th Day of the Year
240 has more divisors (20 of them) than any previous number. What would be the next number that has more? (Yet the number before it, and after it are both prime!)
240 can be expessed as a 3x3 magic square by multiplying each of the basic sauares by 16, or with consecutive numbers from 80 to 89.
Because 240 is divisible by 20 (the number of its divisors, it is called refactorable. And since it is divisible by the sum of its digits, it is called a Harshad or Joy-Giver Number.
240 is the smallest number expressible as the sum of consecutive primes in three ways, *Prime Curios (113+127, 53+59+61+67, 17 + 19 + 23 + 29 + 31 + 37 + 41 + 43.)
240 is the product of the first 6 Fibonacci numbers, 240 = 1*1*2*3*5*8 *Derek Orr
These are often called Fibonacci factorials or fibonorials. 240 would be \(6!_F\), also called the Fibonacci factorial
The Kissing Number, the number of spheres that can be placed around a central sphere so that they all are touching it, for the eighth dimension is 240. Beyond the fourth dimension, only the eighth and twenty-fourth are known exactly. The 24th dimension is the highest dimension for which the exact "kissing number", is known. For the 24th dimension, the "kissing number is 196,560.
For those of you who remember Piet Hein's Soma Cube puzzle ("CAn you solve it? Soma Can, Soma Can't!") there are 240 different solutions.
The Datsun 240 Z was called the "Fairlady Z" in Japan. My son owned one (very used, and maintained by himself and his brother from two more scraped versions) while he was in college.
Because it has so many factors of two, it is expressible as the difference of two squares in several ways, \(240= 61^2 - 59^2 = 32^2 - 28^2 =19^2 - 11^2\)
232 is sum of the cubes of the factorials of its digits,
232 = (2!)3 + (3!)3 + (2!)3
and 232 the sum of the first 11 Fibonacci numbers. 232 = 1+1+2+3+5+8+13+21+34+55+89
If you add up all the proper divisors of a number, n, they can be less than n, as 231 is(deficient), equal to n (perfect, like 6 or 28) or abundant. 12 is the smallest abundant number. Nicomachus wrote only of even numbers because he thought all odd numbers were deficient, but he was wrong. The 232nd abundant number is odd, 945.
If you raise 232 to the power of the product of its digits, and then add the sum of its digits, you get a prime. The only other known number with this property is 187. *Prime Curios
232 /4 = 58, so 59^2 - 57^2 = 232. 232 / 8 = 29 so 31^2-27^2 = 232.
Because 58 is a sum of two squares, 232 is also. 58 =7^2 + 3^2, 232 = 14^2 + 6^2. Students might use this to find the sum of two squares for 522 = 9 x 58.
232 is another balanced binary, with four of each ones and zeros, and always the number of ones is greater than, or equal to, the number of zeros.
232 is one less than a Fibonacci Prime.
The 233rd Day of the Year
233 is the only three digit prime that is also a Fibonacci number. It is the only known Fibonacci prime whose digits are all Fibonacci primes, and the sum of its digits is also a Fibonacci number. *Prime Curios
233 is also the last day of the year that is the sum of the squares of consecutive Fibonacci numbers. (A pretty mathematical fact for the day: the sum of the squares of two consecutive Fibonacci numbers is always a Fibonacci number, Students can show that the converse is not true.) If you square 8 (F6) and add the square of 13(F7) you get 233 (F(6+7))
There are exactly 233 maximal planar graphs with ten vertices, and 233 connected topological spaces with four points.
The ratio of 233/144, two Fibonacci numbers is a good approximation to Phi, the Golden Ratio.
233 is the smallest prime factor of |(2^{29} - 1 \) *Prime Curios
If you start the "see and say" sequence with 233 (a prime), you get 1223, another prime. And if you do it again with 1233, you get another. *Prime Curios
233 is the smallest magic constant of a 5x5 prime Magic square. It uses all the odd primes below 100. *Prime Curios
233 is the sum of the squares of the first four semi-primes, 4^2 + 6^2 + 9^2 + 10^2.
233= 117^2 - 116^2
233 is the sum of eleven consecutive primes, 5 + 7 + 11 + ... + 41
233 is a palindrome in base 3 (22122)
233 in Roman numerals uses 2 C's, 3 X's, and 3 I's. One of the Roman Numeral displays that you can find digital root by counting the number of symbols. Any number using only M, C, X and I will also work
The 234th Day of the Year
234 begins a string of eight consecutive digits which is a prime number, 23456789. All the children need to know why there can not be any ordering of all nine digits , 1 to 9 that is prime. Teach one today. Casting out nines is as old as Iamblichus, and as new as the youngest kid entering kindegarten.
234 is the 55th abundant number. It's proper divisors are {1, 2, 3, 6, 9, 13, 18, 26, 39, 78, 117} and their sum is greater than 234, it is 312. There are 86 abundant numbers in a non-leap year. 234=6(39) and all multiples two or greater of a perfect number are abundant.
9^2 + 6^2 = 117. Can you see how that tells you that 234 = 3^2 + 15^2???
234 is the number of ways to partition 50 intoat most three parts.
The 235th Day of the Year
235 is the number of trees with 11 vertices. (Counting the number of unlabeled free trees is still an open problem in math. No closed formula for the number of trees with n vertices up to graph isomorphism is known.)
If you build an equilateral triangle with nine matchsticks on each side, then subdivide into additional equilateral triangles, there will be a total of 235 triangles of several different sizes. The image shows the subdivision of a equilateral triangle with three matchsticks on a side. Can you find the thirteen triangles in it?
235 is a semi-prime (5 x 47) that is the concatenation of the first three Fibonacci primes
235 is a palindrome in base 4 (32234), 7 (4547), and 8 (3538),
U-235 is the fissile isotope of uranium used in the first atomic bombs.
235, like all numbers ending in 5 that are greater than 25, is the difference of two squares of integers that differ by five, 26^2 - 21^2 = 235. Like all odd numbers, it is the difference of two consecutive squares, 235 = 118^2 - 117^2.
235 is the tenth Heptagonal (7-gon) number. n*(5n-3)/2 where n =10
The 236th Day of the Year
236 is the sum of twelve consecutive primes, 3 + 5 + 7 + 11 + 13 + 17 + 19 + 23 + 29 + 31 + 37 + 41
And 236 is the number of possible positions in Othello after 2 moves by both players. *Erich Friedman (Students might try to figure out how many possible positions are there in tic-tac-toe after 2 moves by each player. Entice them with this number fact from Cliff Pickover @pickover )
The sum of the divisors of 236, 2+2 + 59 =63, the product of it's digits is 2x3x6 = 36, the reversal of the sum of the divisors.*Prime Curios
236^2 + 1 is a prime
236 is the average of two consecutive primes 233 and 239.
236 is a Happy Number. The sum of the squares of the digits under iteration go to 1.
Since 236/4 = 59, 236 = 60^2 - 58^2.
The 237th Day of the Year
it would be a singularly uninteresting number (3 x 79) except that the room number in the film, "The Shining" was switched from 217 in the novel to 237 for the film? It seems that the Timberline Lodge had a room 217 but no room 237, so the hotel management asked Kubrick to change the room number because they were afraid their guests might not want to stay in room 217 after seeing the film. *Visual Memory.co.uk
Derek Orr added, 237 = 44th prime + 44 = 193 + 44 What's the next number that equals the n-th prime + n?
237 is the difference of two consecutive squares, like all odd integers, 119^2 - 118^2 = 237, but also 41^2 - 38^2 because 6*38+9 = 237.
The 237th square pyramidal number, 4465475, is also a sum of two smaller square pyramidal numbers. There are only four smaller numbers (55, 70, 147, and 226) with the same property. *Wikipedia
237 is a lucky number, it remains after all sieves in the Eratosthenes-like Sieve of Stan Ulam. " Start with the natural numbers. Delete every 2nd number, leaving 1 3 5 7 ...; the 2nd number remaining is 3, so delete every 3rd number, leaving 1 3 7 9 13 15 ...; now delete every 7th number, leaving 1 3 7 9 13 ...; now delete every 9th number; etc." *OEIS
237 is the last of three consecutive odd numbers that have all prime digits.
237 not only has all prime digits, any substring of 2 consecutive digits also form a prime, 23, 37.
The 238th Day of the Year
The 238th day of the year; 238 is an untouchable number, The untouchable numbers are those that are not the sum of the proper divisors of any number. 2 and 5 are untouchable, can you find the next one? (four is not untouchable, for example since 1+3=4 and they are the proper divisors of 9) Five is the only known odd untouchable number.
238 is also the sum of the first 13 primes, and its digits add up to ........wait for it.... 13 (2+3+8 = 13 and 238 = sum of first 13 primes). Only two more year dates is the sum of the first n primes.
23=8 (We are tentatively calling these "power equation numbers") *Derek Orr
In base 16 238 is a Repdigit, EE (14 x 16 + 14
238 is the number of partitions of 34 into powers of two.And also the same for 35.
6 times 238 is between a pair of twin primes.
The 239th Day of the Year
When expressing 239 as a sum of square numbers, 4 squares are required, which is the maximum that any integer can require; it is the largest number that needs the maximum number (9) of positive cubes. 239 = 5^3 + 3^3 + 3^3 + 3^3 + 2^3 + 2^3 + 2^3 + 2^3 + 1^3. (Only one other number requires nine cubes, can you find it?)
and a hundred years (+/-) ago (many people included 1 as a prime then; see more) 239 would have been a prime that is the sum of the first 14 primes; 239 = 1+2+3+5+7+11+...+37+41 *Derek Orr
The sum of the odd numbers from 1 to 239 is equal to the sum of the odd numbers from 239 to 337.
239 appears in one of the earliest known geometrically converging formulas for computing Pi: Pi/4 = 4 arctan(1/5) - arctan(1/239) *.archimedes-lab.org
239 is the 52nd prime, and the smaller of a pair of twin primes with 241.
For any number, greater than 239, the largest factor of n^2 + 1 is at least 17. *Prime Curios
If you factor 1234567654321, the smallest prime factor is 239. *Prime Curios
Arctan(1/239) in degrees begins 0.239..., and this is the only positive integer for which this is true. *Prime Curios
The 52nd prime number (239) has a Collatz trajectory length of 52. It is also the smallest integer greater than 1 that has the same Collatz trajectory length as its square (57121) *Prime Cuiros
There are 239 primes less than 1500.
239/169 is a convergent of the continued fraction of the square root of 2, so is is a solution to the Pell Equation 239^2 = 2 · 169^2 − 1.
The only solutions of the Diophantine equation y^2 + 1 = 2x^4 in positive integers are (x, y) = (1, 1) or (13, 239).*Wikipedia
The 240th Day of the Year
240 has more divisors (20 of them) than any previous number. What would be the next number that has more? (Yet the number before it, and after it are both prime!)
240 can be expessed as a 3x3 magic square by multiplying each of the basic sauares by 16, or with consecutive numbers from 80 to 89.
Because 240 is divisible by 20 (the number of its divisors, it is called refactorable. And since it is divisible by the sum of its digits, it is called a Harshad or Joy-Giver Number.
240 is the smallest number expressible as the sum of consecutive primes in three ways, *Prime Curios (113+127, 53+59+61+67, 17 + 19 + 23 + 29 + 31 + 37 + 41 + 43.)
240 is the product of the first 6 Fibonacci numbers, 240 = 1*1*2*3*5*8 *Derek Orr
These are often called Fibonacci factorials or fibonorials. 240 would be \(6!_F\), also called the Fibonacci factorial
The Kissing Number, the number of spheres that can be placed around a central sphere so that they all are touching it, for the eighth dimension is 240. Beyond the fourth dimension, only the eighth and twenty-fourth are known exactly. The 24th dimension is the highest dimension for which the exact "kissing number", is known. For the 24th dimension, the "kissing number is 196,560.
For those of you who remember Piet Hein's Soma Cube puzzle ("CAn you solve it? Soma Can, Soma Can't!") there are 240 different solutions.
The Datsun 240 Z was called the "Fairlady Z" in Japan. My son owned one (very used, and maintained by himself and his brother from two more scraped versions) while he was in college.
Because it has so many factors of two, it is expressible as the difference of two squares in several ways, \(240= 61^2 - 59^2 = 32^2 - 28^2 =19^2 - 11^2\)






No comments:
Post a Comment